If you’re studying calculus or advanced mathematics, chances are you’ve encountered the p series test. This essential concept is a cornerstone in understanding series convergence and plays a pivotal role in solving infinite series problems. Whether you’re a student preparing for an exam, a tutor looking to explain the concept, or simply a math enthusiast, mastering the P-Series Test is crucial.
This comprehensive guide will cover everything you need to know about the P-Series Test, including its definition, applications, and examples, along with tips for solving related problems. Let’s dive in!
What is the P-Series Test?
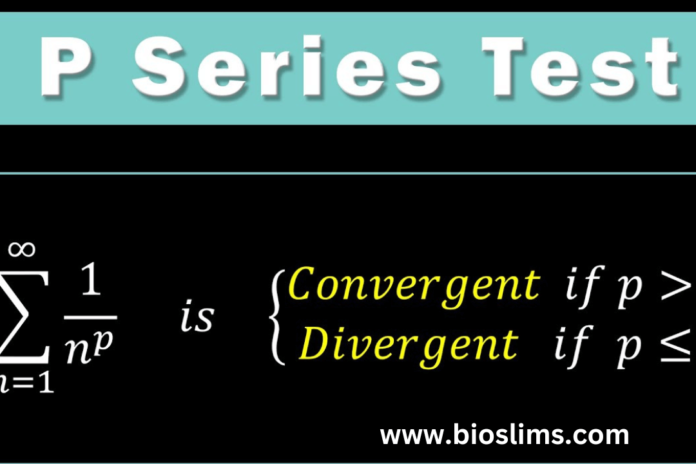
The p series test is a mathematical method used to determine the convergence or divergence of a series in the form:
Where is a constant? The behaviour of the series depends entirely on the Value of the following:
- If the series converges.
- If the series diverges.
This simple yet powerful test is widely used in calculus and analysis to analyze infinite series.
Why is the P-Series Test Important?
Understanding the p series test is critical for several reasons:
- Foundation for Infinite Series: The test provides an easy way to analyze a common type of infinite series, forming the basis for more complex convergence tests.
- Applications in Calculus: The P-Series Test is used to solve integral problems, estimate errors, and analyze functions.
- Practical Relevance: Infinite series, including p-series, are used in physics, engineering, and computer science to model real-world phenomena.
- Exam Preparation: If you’re studying advanced math, knowing the P-Series Test is essential for tackling questions on series convergence.
Breaking Down the P-Series Test
Let’s explore the two cases of the p series test in detail:
- Case 1: Convergence ()
When, the series:
Converges. This means that as you add more and more terms, the sum approaches a finite value.
Why Does it Converge?
For, the terms decrease rapidly enough to make the sum finite. This behaviour can be proven using comparison tests or integral tests.
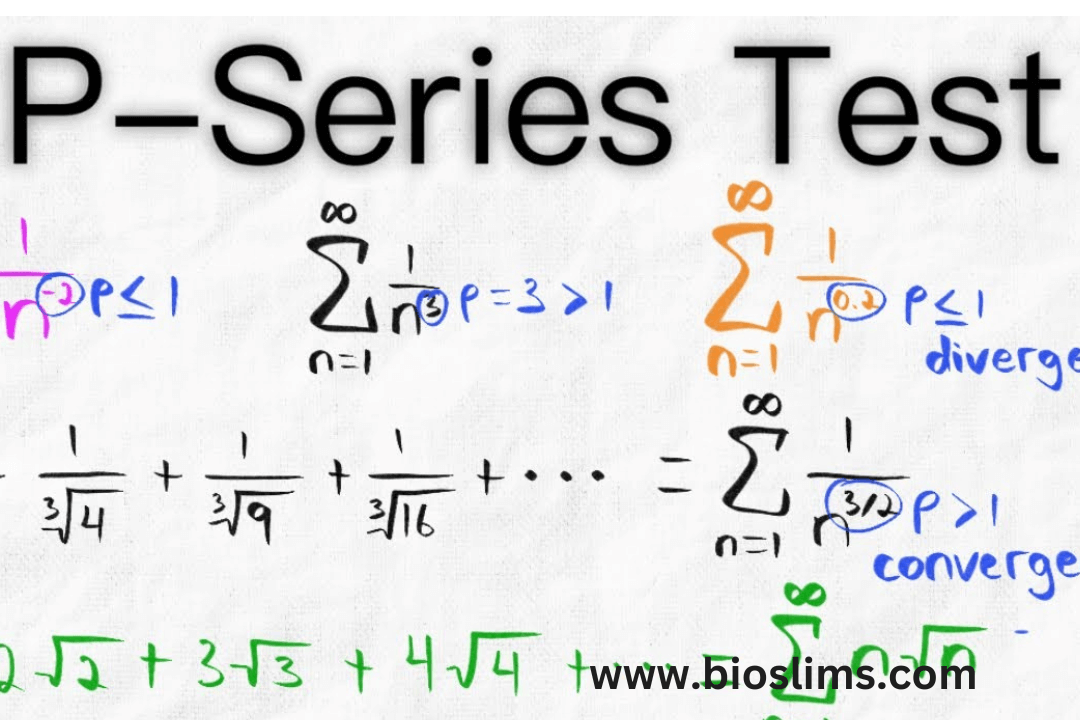
Example:
Here, Using the P-Series Test, we conclude that the series converges. The sum of this series is known as the Basel problem result, equal to.
- Case 2: Divergence ()
When the series diverges, this means that as you add more terms, the sum grows without bound or fails to approach a finite value.
Why Does it Diverge?
For, the terms do not decrease quickly enough to result in a finite sum. In fact, the series becomes the harmonic series, which is well-known to diverge.
Example:
Here, Using the P-Series Test, we determine that the series diverges. This is the famous harmonic series, which grows without bounds.
Applications of the P-Series Test
The P-Series Test is more than just a theoretical tool; it has practical applications across various fields. Here’s where you might encounter it:
- Evaluating Series in Calculus
When solving problems involving infinite series, the P-Series Test is often the first method used to determine convergence.
- Physics and Engineering
Infinite series, including p-series, are used in modelling waveforms, heat conduction, and other physical phenomena.
- Computer Science
Series convergence plays a role in algorithms, numerical analysis, and even machine learning techniques.
- Error Estimation
In numerical methods, the convergence of series is crucial for estimating errors and improving accuracy.
How to Use the P-Series Test
Here’s a step-by-step guide to applying the P-Series Test:
- Identify the Series: Ensure the series is in the form.
- Determine the valueValue of: Look at the exponent in the denominator.
- Apply the Test:
- If the series converges.
- If the series diverges.
- Justify Your Answer: Use reasoning or additional tests (if needed) to support your conclusion.
Common Mistakes to Avoid
When using the P-Series Test, students often make the following mistakes. Here’s how to avoid them:
- Misidentifying the Form
Ensure the series matches the p-series format if it doesn’t, consider other convergence tests like the Comparison Test or Ratio Test.
- Confusing with Other Variables
The Value must be constant. If the exponent changes, the series is not a p-series.
- Ignoring the Behavior of Terms
Remember, convergence depends on the rate of decrease of terms. Carefully analyze how it behaves as it increases.
Examples and Practice Problems
Let’s solidify your understanding of the P-Series Test with examples and practice problems:
Example 1: Convergent Series
Does the series converge?
Solution:
- Here,
- By the P-Series Test, the series converges.
Example 2: Divergent Series
Does the series converge?
Solution:
- Here,
- By the P-Series Test, the series diverges.
Practice Problems
- Determine if the series converges or diverges.
- Prove whether the harmonic series diverges using the P-Series Test.
- Analyze the convergence of.
Tips for Mastering the P-Series Test
To excel in applying the P-Series Test, keep these tips in mind:
- Practice Regularly: Solve a variety of problems to build confidence.
- Understand the Theory: Know why the test works, not just how to use it.
- Combine with Other Tests: Use the P-Series Test alongside other convergence tests for complex problems.
- Stay Organized: Write out each step clearly to avoid mistakes.
Final Thoughts
The P-Series Test is a fundamental tool for analyzing the convergence of infinite series. Its simplicity and effectiveness make it a favourite among students and professionals alike. By understanding the conditions for convergence and divergence, you can tackle a wide range of mathematical problems with confidence.
Whether you’re preparing for an exam, solving real-world problems, or exploring advanced mathematics, mastering the P-Series Test will serve you well. Practice regularly, stay curious, and enjoy the journey of learning this essential mathematical concept!
You may also read: Exploring R Poe: A Comprehensive Guide to a Rising Trend